Alex’s Adventures in Numberland (59 page)

IGT’s most popular progressive slot, Megabucks, links together hundreds of machines across Nevada. When the company introduced it a decade ago, the minimum jackpot was $1 million. Initially, casinos didn’t want the liability of having to pay out so much, so IGT underwrote the entire network by taking a percentage from all of its machines, and paid the jackpot itself. Despite paying out hundreds of millions of dollars in prize money, IGT has never suffered a loss on Megabucks. The law of large numbers is remarkably reliable: the bigger you get, the better it all works out.

The Megabucks jackpot now starts at $10 million. If it hasn’t been won by the time the jackpot reaches around $20 million, casinos see queues forming at their Megabucks slots and IGT gets requests to distribute more machines. ‘People think it’s past that point where i normally hits so it’s going to hit soon,’ explained Baerlocher.

This reasoning, however, is erroneous. Every game played on a slot machine is a random event. You are just as likely to win when the jackpot is at $10, $20 or even $100 million, but it is very instinctive to feel that after a long period of holding money back, the machines are more likely to pay out. The belief that a jackpot is ‘due’ is known as the
gambler’s fallacy
.

The gambler’s fallacy is an incredibly strong human urge. Slot machines tap into it with particular virulence, which makes them, perhaps, the most addictive of all casino games. If you are playing many games in quick succession, it is only natural to think after a long run of losses: ‘I’m bound to win next time.’ Gamblers often talk of a machine being ‘hot’ or ‘cold’ – meaning that it is paying out lots or paying out little. Again, this is nonsense, since the odds are always the same. Still, one can see why one might attribute personality to a human-sized piece of plastic and metal often referred to as a one-armed bandit. Playing a slot machine is an intense, intimate experience – you get right up close, tap it with your fingertips, and cut out the rest of the world.

Because our brains are bad at understanding randomness, probability is the branch of maths most riddled with paradoxes and surprises. We instinctively attribute patterns to situations, even when we know there are none. It’s easy to be dismissive of a slot-machine player for thinking that a machine is more likely to pay out after a losing streak, yet the psychology of the gambler’s fallacy is present in non-gamblers too.

Consider the following party trick. Take two people, and explain to them that one will flip a coin 30 times and write down the order of heads and tails. The other will imagine flipping a coin 30 times, and then will write down the order of heads and tails outcomes that they have visualized. Without telling you, the two players will decide who takes which role, and then present you with their two lists. I asked my mother and stepfather to do this and was handed the following:

List 1

H T T H T H T T T H H T H H T H H H H T H T T H T H T T H H

List 2

T T H H T T T T T H H T T T H T T H T H H H H T H H T H T H

The point of this game is that it is very easy to spot which list comes from the flipping of the real coin, and which from the imagined coin. In the above case, it was clear to me that the second list was the real one, which was correct. First, I looked at the maximum runs of heads or tails. The second list had a maximum run of 5 tails. The first list had a maximum run of 4 heads. The probability of a run of 5 in 30 flips is almost two thirds, so it is much more likely than not that 30 flips gives a run of 5. The second list was already a good candidate for the real coin. Also, I knew that most people never ascribe a run of 5 in 30 flips because it seems too deliberate to be random. But to be sure I was right that the second list was the real coin I looked at how frequently both lists alternated between heads and tails. Due to the fact that each time you flip a coin the chances of heads and tails are equal, you would expect each outcome to be followed by a different outcome about half the time, and half the time to be followed by the same outcome. The second list alternates 15 times. The first list alternates 19 times – evidence of human interference. When imagining coin flips, our brains tend to alternate outcomes much more frequently than what actually occurs in a truly random sequence – after a couple of heads, our instinct is to compensate and imagine an outcome of tails, even though the chance of heads is still just as likely. Here, the gambler’s fallacy appears. True randomness has no memory of what came before.

The human brain finds it incredibly difficult, if not impossible, to fake randomness. And when we are presented with randomness, we often interpret it as non-random. For example, the shuffle feature on an iPod plays songs in a random order. But when Apple launched the feature, customers complained that it favoured certain bands because often tracks from the same band were played one after another. The listeners were guilty of the gambler’s fallacy. If the iPod shuffle were truly random, then each new song choice is independent of the previous choice. As the coin-flipping experiment shows, counterintuitively long streaks are the norm. If songs are chosen randomly, it is very possible, if not entirely likely, that there will be clusters of songs by the same artist. Apple CEO Steve Jobs was totally serious when he said, in response to the outcry: ‘We’re making [the shuffle] less random to make it feel more random.’

Why is the gambler’s fallacy such a strong human urge? It’s all about control. We like to feel in control of our environments. If events occur randomly, we feel that we have no control over them. Conversely, if we do have control over events, they are not random. This is why we prefer to see patterns when there are none. We are trying to salvage a feeling of control. The human need to be in control is a deep-rooted survival instinct. In the 1970s a fascinating (if brutal) experiment examined how important a sense of control was for elderly patients in a nursing home. Some patients were allowed to choose how their rooms were arranged and allowed to choose a plant to look after. The others were told how their rooms would be and had a plant chosen and tended for them. The result after 18 months was striking. The patients who had control over their rooms had a 15 percent death rate, but for those who had no control the rate was 30 percent. Feeling in control can keep us alive.

Randomness is not smooth. It creates areas of empty space and areas of overlap.

Random dots; non-random dots.

Randomness can explain why some small villages have higher than normal rates of birth defects, why certain roads have more accidents, and why in some games basketball players seem to score every free throw. It’s also why in seven of the last ten World Cup finals at least two players shared birthdays:

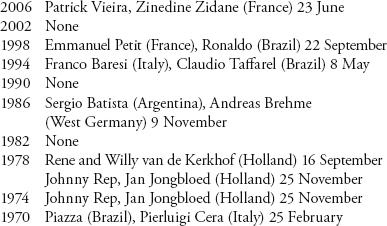
While at first this seems like an amazing series of coincidences, the list is actually mathematically unsurprising because whenever you have a randomly selected group of 23 people (such as two football teams and a referee), it is more likely than not that two people in it will share the same birthday. The phenomenon is known as the ‘birthday paradox’. There is nothing self-contradictory about the result, but it does fly in the face of common sense – twenty-three seems like an absurdly small number.

Proof of the birthday paradox is similar to the proofs we used at the beginning of this chapter for rolling certain combinations of dice. In fact, we could rephrase the birthday paradox as the statement that for a 365-sided dice, after 23 throws it will be more likely than not that the dice will have landed on the same side twice.

Football matches provide the perfect sample group to see if the facts fit the theory because there are always 23 people on the pitch. Looking at World Cup finals, however, the birthday paradox works a little too well. The probability of two people having the same birthday in a group of 23 is 0.507 or just over 50 percent. Yet with seven out of ten positives (even excluding the van de Kerkhof twins), we have achieved a 70 percent strike rate.