Alex’s Adventures in Numberland (2 page)
Read Alex’s Adventures in Numberland Online
Authors: Alex Bellos


When I walked into Pierre Pica’s cramped Paris apartment I was overwhelmed by the stench of mosquito repellent. Pica had just returned from spending five months with a community of Indians in the Amazon rainforest, and he was disinfecting the gifts he had brought back. The walls of his study were decorated with tribal masks, feathered headdresses and woven baskets. Academic books overloaded the shelves. A lone Rubik’s Cube lay unsolved on a ledge.
I asked Pica how the trip had been.
‘Difficult,’ he replied.
Pica is a linguist and, perhaps because of this, speaks slowly and carefully, with painstaking attention to individual words. He is in his fifties, but looks boyish – with bright blue eyes, a reddish complexion and soft, dishevelled silvery hair. His voice is quiet; his manner intense.
Pica was a student of the eminent American linguist Noam Chomsky and is now employed by France’s National Centre for Scientific Research. For the last ten years the focus of his work has been the Munduruku, an indigenous group of about 7000 people in the Brazilian Amazon. The Munduruku are hunter-gatherers who live in small villages spread across an area of rainforest twice the size of Wales. Pica’s interest is the Munduruku language: it has no tenses, no plurals and no words for numbers beyond five.
To undertake his fieldwork, Pica embarks on a journey worthy of the great adventurers. The nearest large airport to the Indians is Santarém, a town 500 miles up the Amazon from the Atlantic Ocean. From there, a 15-hour ferry ride takes him almost 200 miles along the Tapajós River to Itaituba, a former gold-rush town and the last stop to stock up on food and fuel. On his most recent trip Pica hired a jeep in Itaituba and loaded it up with his equipment, which included computers, solar panels, batteries, books and 120 gallons of petrol. Then he set off down the Trans-Amazon Highway, a 1970s folly of nationalistic infrastructure that has deteriorated into a precarious and often impassable muddy track.
Pica’s destination was Jacareacanga, a small settlement a further 200 miles southwest of Itaituba. I asked him how long it took to drive there. ‘Depends,’ he shrugged. ‘It can take a lifetime. It can take two days.’
How long did it take
this
time, I repeated.
‘You know, you never know how long it will take because it never takes the same time. It takes between ten and twelve hours during the rainy season. If everything goes well.’
Jacareacanga is on the edge of the Munduruku’s demarcated territory. To get inside the area, Pica had to wait for some Indians to arrive so he could negotiate with them to take him there by canoe.
‘How long did you wait?’ I enquired.
‘I waited quite a lot. But, again, don’t ask me how many days.’
‘So, it was a couple of days?’ I suggested tentatively.
A few seconds passed as he furrowed his brow. ‘It was about two weeks.’
More than a month after he left Paris, Pica was finally approaching his destination. Inevitably, I wanted to know how long it took to get from Jacareacanga to the villages.
But by now Pica was demonstrably impatient with my line of questioning: ‘Same answer to everything –
it depends!
’
I stood my ground. How long did it take
this
time?
He stuttered: ‘I don’t know. I think…perhaps…two days…a day and a night…’
The more I pushed Pica for facts and figures, the more reluctant he was to provide them. I became exasperated. It was unclear if underlying his responses was French intransigence, academic pedantry or simply a general contrariness. I stopped my line of questioning and we moved on to other subjects. It was only when, a few hours later, we talked about what it was like to come home after so long in the middle of nowhere that he opened up. ‘When I come back from Amazonia I lose sense of time and sense of number, and perhaps sense of space,’ he said. He forgets appointments. He is disoriented by simple directions. ‘I have extreme difficulty adjusting to Paris again, with its angles and straight lines.’ Pica’s inability to give me quantitative data was part of his culture shock. He had spent so long with people who can barely count that he had lost the ability to describe the world in terms of numbers.
No one knows for certain, but numbers are probably no more than about 10,000 years old. By this I mean a working system of words and symbols for numbers. One theory is that such a practice emerged together with agriculture and trade, as numbers were an indispensable tool for taking stock and making sure you were not ripped off. The Munduruku are only subsistence farmers and money has only recently begun to circulate in their villages, so they never evolved counting skills. In the case of the indigenous tribes of Papua New Guinea, it has been argued that the appearance of numbers was triggered by elaborate customs of gift exchange. The Amazon, by contrast, has no such traditions.
Tens of thousands of years ago, well before the arrival of numbers, however, our ancestors must have had certain sensibilities about amounts. They would have been able to distinguish one mammoth from two mammoths, and to recognize that one night is different from two nights. The intellectual leap from the concrete idea of two things to the invention of a symbol or word for the abstract idea of ‘two’, however, will have taken many ages to come about. This occurrence, in fact, is as far as some communities in the Amazon have come. There are tribes whose only number words are ‘one’, ‘two’ and ‘many’. The Munduruku, who go all the way up to five, are a relatively sophisticated bunch.
Numbers are so prevalent in our lives that it is hard to imagine how people survive without them. Yet while Pierre Pica stayed with the Munduruku he easily slipped into a numberless existence. He slept in a hammock. He went hunting and ate tapir, armadillo and wild boar. He told the time from the position of the sun. If it rained, he stayed in; if it was sunny, he went out. There was never any need to count.
Still, I thought it odd that numbers larger than five did not crop up at all in Amazonian daily life. I asked Pica how an Indian would say ‘six fish’. For example, just say that he or she was preparing a meal for six people and he wanted to make sure everyone had a fish each.
‘It is impossible,’ he said. ‘The sentence “I want fish for six people” does not exist.’
What if you asked a Munduruku who had six children: ‘How many kids do you have?’
Pica gave the same response: ‘He will say “I don’t know”. It is impossible to express.’
However, added Pica, the issue was a cultural one. It was not the case that the Munduruku counted his first child, his second, his third, his fourth, his fifth and then scratched his head because he could go no further. For the Munduruku, the whole idea of counting children was ludicrous. The whole idea, in fact, of counting anything was ludicrous.
Why would a Munduruku adult want to count his children, asked Pica? The children are looked after by all the adults in the community, he said, and no one is counting who belongs to whom. He compared the situation to the French expression
‘ j’ai une grande famille’
, or ‘I’m from a big family’. ‘When I say that I have a big family I am telling you that I don’t know [how many members it has]. Where does my family stop and where does the others’ family begin? I don’t know. Nobody ever told me that.’ Similarly, if you asked an adult Munduruku how many children he is responsible for, there is no correct answer. ‘He will answer “I don’t know”, which really is the case.’
The Munduruku are not alone in the sweep of history in not counting members of their community. When King David counted his own people he was punished with three days of pestilence and 77,000 deaths. Jews are meant to count Jews only indirectly, which is why in synagogues the way of making sure there are ten men present, a
minyan
, or sufficient community for prayers, is to say a ten-word prayer pointing at each person per word. Counting people with numbers is considered a way of singling people out, which makes them more vulnerable to malign influences. Ask an Orthodox rabbi to count his kids and you have as much chance of an answer as if you asked a Munduruku.
I once spoke to a Brazilian teacher who had spent a lot of time working in indigenous communities. She said that Indians thought that the constant questioning by outsiders of how many children they had was a peculiar compulsion, even though the visitors were simply asking the question to be polite. What is the purpose of counting children? It made the Indians very suspicious, she said.
um, dois, três,
up until the hundreds,’ said Pica. ‘Then you ask them, “By the way, how much is five minus three?”’ He parodied a Gallic shrug. They have no idea.
In the rainforest Pica conducts his research using laptops powered by solar-charged batteries. Maintaining the hardware is a logistical nightmare because of the heat and the damp, although sometimes the trickiest challenge is assembling the participants. On one occasion the leader of a village demanded that Pica eat a large, red
sauba
ant in order to gain permission to interview a child. The ever-diligent linguist grimaced as he crunched the insect and swallowed it down.
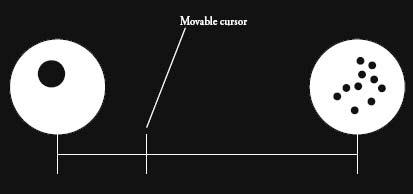
The purpose of researching the mathematical abilities of people who have the capacity to count only on one hand is to discover the nature of our basic numerical intuitions. Pica wants to know what is universal to all humans, and what is shaped by culture. In one of his most fascinating experiments he examined the Indians’ spatial understanding of numbers. How did they visualize numbers when spread out on a line? In the modern world we do this all the time – on tape measures, rulers, graphs and houses along a street. Since the Munduruku don’t have numbers, Pica tested them using sets of dots on a screen. Each volunteer was presented with the figure overleaf, of an unmarked line. To the left side of the line was one dot; to the right, ten dots. Each volunteer was then shown random sets of between one and ten dots. For each set the subject had to point at where on the line he or she thought the number of dots should be located. Pica moved the cursor to this point and clicked. Through repeated clicks, he could see exactly how the Munduruku spaced numbers between one and ten.
When American adults were given this test, they placed the numbers at equal intervals along the line. They recreated the number line we learn at school, in which adjacent digits are the same distance apart as if measured by a ruler. The Munduruku, however, responded quite differently. They thought that intervals between the numbers started large and became progressively smaller as the numbers increased. For example, the distance between the marks for one dot and two dots, and two dots and three dots were much larger than the distance between seven and eight dots, or eight and nine dots, as the following two graphs make clear.
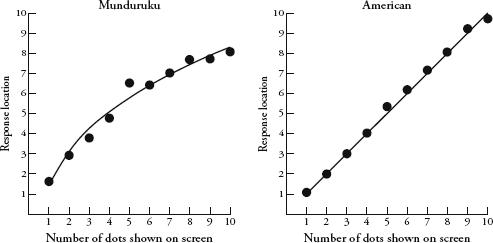
The results were striking. It is generally considered a self-evident truth that numbers are evenly spaced. We are taught this at school and we accept it easily. It is the basis of all measurement and science. Yet the Munduruku do not see the world like this. Stripped of a language of counting and number words, theyvisualize magnitudes in a completely different way.
When numbers are spread out evenly on a ruler, the scale is called
linear
. When numbers get closer as they get larger, the scale is called
logarithmic
.
*
It turns out that the logarithmic approach is not exclusive to Amazonian Indians. We are all born conceiving numbers this way. In 2004, Robert Siegler and Julie Booth at Carnegie Mellon University in Pennsylvania presented a similar version of the number-line experiment to a group of kindergarten pupils (with an average age of 5.8 years), first-graders (6.9) and second-graders (7.8). The results showed in slow motion how familiarity with counting moulds our intuitions. The kindergarten pupil, with no formal maths education, maps out numbers logarithmically. By the first year at school, when the pupils are being introduced to number words and symbols, the curve is straightening. And by the second year at school, the numbers are at last evenly laid out along the line.

Why do Indians and children think that higher numbers are closer together than lower numbers? There is a simple explanation. In the experiments, the volunteers were presented with a set of dots and asked where this set should be located in relation to a line with one dot on the left and ten dots on the right. (Or, in the children’s case, 100 dots). Imagine a Munduruku is presented with five dots. He will study it closely and see that five dots are
five times bigger
than one dot, but ten dots are only
twice as big
as five dots. The Munduruku and the children seem to be making their decisions about where numbers lie based on estimating the ratios between amounts. When considering ratios, it is logical that the distance between five and one is much greater than the distance between ten and five. And if you judge amounts using ratios, you will always produce a logarithmic scale.
It is Pica’s belief that understanding quantities approximately in terms of estimating ratios is a universal human intuition. In fact, humans who do not have numbers – like Indians and young children – have no alternative but to see the world in this way. By contrast, understanding quantities in terms of exact numbers is not a universal intuition; it is a product of culture. The precedence of approximations and ratios over exact numbers, Pica suggests, is due to the fact that ratios are much more important for survival in the wild than the ability to count. Faced with a group of spear-wielding adversaries, we needed to know instantly whether there were more of them than us. When we saw two trees we needed to know instantly which had more fruit hanging from it. In neither case was it necessary to enumerate every enemy or every fruit individually. The crucial thing was to be able to make quick estimates of the relevant amounts and compare them, in other words to make approximations and judge their ratios.
The logarithmic scale is also faithful to the way distances are perceived, which is possibly why it is so intuitive. It takes account of perspective. For example, if we see a tree 100m away and another 100m behind it, the second 100m looks shorter. To a Munduruku, the idea that every 100m represents an equal distance is a distortion of how he perceives the environment.
Exact numbers provide us with a linear framework that contradicts our logarithmic intuition. Indeed, our proficiency with exact numbers means that the logarithmic intuition is overruled in most situations. But it is not eliminated altogether. We live with both a linearand a logarithmic understanding of quantity. For example, our understanding of the passing of time tends to be logarithmic. We often feel that time passes faster the older we get. Yet it works in the other direction too: yesterday seems a lot longer than the whole of last week. Our deep-seated logarithmic instinct surfaces most clearly when it comes to thinking about very large numbers. For example, we can all understand the difference between one and ten. It is unlikely we would confuse one pint of beer and ten pints of beer. Yet what about the difference between a billion gallons of water and ten billion gallons of water? Even though the difference is enormous, we tend to see both quantities as quite similar – as very large amounts of water. Likewise, the terms millionaire and billionaire are thrown around almost as synonyms – as if there is not so much difference between being very rich and very, very rich. Yet a billionaire is a thousand times richer than a millionaire. The higher numbers are, the closer together they feel.
The fact that Pica temporarily forgot how to use numbers after only a few months in the jungle indicates that our linear understanding of numbers is not as deeply rooted in our brains as our logarithmic one. Our understanding of numbers is surprisingly fragile, which is why without regular use we lose our ability to manipulate exact numbers and default to our intuitions judging amounts with approximations and ratios.
Pica said that his and others’ research on our mathematical intuitions may have serious consequences for maths education – both in the Amazon and in the West. We require understanding of the linear number line to function in modern society – it is the basis of measuring, and facilitates calculations. Yet maybe in our dependence on linearity we have gone too far in stifling our own logarithmic intuition. Perhaps, said Pica, this is a reason why so many people find maths difficult. Perhaps we should pay more attention to judging ratios rather than manipulating exact numbers. Likewise, maybe it would be wrong to teach the Munduruku to count like we do since this might deprive them of the mathematical intuitions or knowledge that are necessary for their own survival.
Interest in the mathematical abilities of those who have no words or symbols for numbers has traditionally focused on animals. One of the best-known research subjects was a trotting stallion called Clever Hans. In the early 1900s, crowds gathered regularly in a Berlin courtyard to watch Hans’s owner, Wilhelm von Osten, a retired maths instructor, set the horse simple arithmetical sums. Hans answered by stamping the ground with his hoof the correct number of times. His repertoire included addition and subtraction as well as fractions, square roots and factorization. Public fascination, and suspicion that the horse’s supposed intelligence was some kind of trick, led to an investigation of his abilities by a committee of eminent scientists. They concluded that,
jawohl!
, Hans really was doing the math.
It took a less eminent but more rigorous psychologist to debunk the equine Einstein. Oscar Pfungst noticed that Hans was reacting to cues in von Osten’s body language. Hans would start stamping his hoof on the ground and stopped only when he could sense a build-up or release of tension in von Osten’s face, indicating the answer had been reached. The horse was sensitive to the tiniest visual signals, such as the leaning of the head, the raising of the eyebrows and even the dilation of the nostrils. Von Osten was not even aware he was making these gestures. Hans was clever at reading people, certainly, but was no arithmetician.
Many further attempts were made in the last century to teach animals to count, not all for the purposes of circus-like entertainment. In 1943 the German scientist Otto Koehler trained his pet raven Jakob to select a pot with a specified number of spots on its lid from a selection of pots with a variety of numbers of spots on their lids. The bird could perform this task when the number of spots on any one lid was between one and seven spots. In recent years, avian intelligence has reached more impressive heights. Irene Pepperberg of Harvard University taught an African grey parrot called Alex numerals from 1 to 6. When shown an assortment of coloured blocks he could reply, for example, how many blue blocks there were by squawking the English number word. So renowned had Alex become among scientists and birdlovers that when he died unexpectedly in 2007, his obituary appeared in
The Economist
.