A Briefer History of Time (2 page)

3

THE NATURE OF A SCIENTIFIC THEORY

IN ORDER TO TALK ABOUT THE nature of the universe and to discuss such questions as whether it has a beginning or an end, you have to be clear about what a scientific theory is. We shall take the simpleminded view that a theory is just a model of the universe, or a restricted part of it, and a set of rules that relate quantities in the model to observations that we make. It exists only in our minds and does not have any other reality (whatever that might mean). A theory is a good theory if it satisfies two requirements. It must accurately describe a large class of observations on the basis of a model that contains only a few arbitrary elements, and it must make definite predictions about the results of future observations. For example, Aristotle believed Empedocles’s theory that everything was made out of four elements: earth, air, fire, and water. This was simple enough but did not make any definite predictions. On the other hand, Newton’s theory of gravity was based on an even simpler model, in which bodies attracted each other with a force that was proportional to a quantity called their mass and inversely proportional to the square of the distance between them. Yet it predicts the motions of the sun, the moon, and the planets to a high degree of accuracy.

Any physical theory is always provisional, in the sense that it is only a hypothesis: you can never prove it. No matter how many times the results of experiments agree with some theory, you can never be sure that the next time a result will not contradict the theory. On the other hand, you can disprove a theory by finding even a single observation that disagrees with the predictions of the theory. As philosopher of science Karl Popper has emphasized, a good theory is characterized by the fact that it makes a number of predictions that could in principle be disproved or falsified by observation. Each time new experiments are observed to agree with the predictions, the theory survives and our confidence in it is increased; but if ever a new observation is found to disagree, we have to abandon or modify the theory.

At least that is what is supposed to happen, but you can always question the competence of the person who carried out the observation.

In practice, what often happens is that a new theory is devised that is really an extension of the previous theory. For example, very accurate observations of the planet Mercury revealed a small difference between its motion and the predictions of Newton’s theory of gravity. Einstein’s general theory of relativity predicted a slightly different motion than Newton’s theory did. The fact that Einstein’s predictions matched what was seen, while Newton’s did not, was one of the crucial confirmations of the new theory. However, we still use Newton’s theory for most practical purposes because the difference between its predictions and those of general relativity is very small in the situations that we normally deal with. (Newton’s theory also has the great advantage that it is much simpler to work with than Einstein’s!)

The eventual goal of science is to provide a single theory that describes the whole universe. However, the approach most scientists actually follow is to separate the problem into two parts. First, there are the laws that tell us how the universe changes with time. (If we know what the universe is like at any one time, these physical laws tell us how it will look at any later time.) Second, there is the question of the initial state of the universe. Some people feel that science should be concerned with only the first part; they regard the question of the initial situation as a matter for metaphysics or religion. They would say that God, being omnipotent, could have started the universe off any way He wanted. That may be so, but in that case God also could have made it develop in a completely arbitrary way. Yet it appears that God chose to make it evolve in a very regular way, according to certain laws. It therefore seems equally reasonable to suppose that there are also laws governing the initial state.

It turns out to be very difficult to devise a theory to describe the universe all in one go. Instead, we break the problem up into bits and invent a number of partial theories. Each of these partial theories describes and predicts a certain limited class of observations, neglecting the effects of other quantities, or representing them by simple sets of numbers. It may be that this approach is completely wrong. If everything in the universe depends on everything else in a fundamental way, it might be impossible to get close to a full solution by investigating parts of the problem in isolation. Nevertheless, it is certainly the way that we have made progress in the past. The classic example is again the Newtonian theory of gravity, which tells us that the gravitational force between two bodies depends only on one number associated with each body, its mass, and is otherwise independent of what the bodies are made of. Thus we do not need to have a theory of the structure and constitution of the sun and the planets in order to calculate their orbits.

Today scientists describe the universe in terms of two basic partial theories—the general theory of relativity and quantum mechanics. They are the great intellectual achievements of the first half of the twentieth century. The general theory of relativity describes the force of gravity and the large-scale structure of the universe; that is, the structure on scales from only a few miles to as large as a million million million million (1 with twenty-four zeros after it) miles, the size of the observable universe. Quantum mechanics, on the other hand, deals with phenomena on extremely small scales, such as a millionth of a millionth of an inch. Unfortunately, however, these two theories are known to be inconsistent with each other—they cannot both be correct. One of the major endeavors in physics today, and the major theme of this book, is the search for a new theory that will incorporate them both—a quantum theory of gravity. We do not yet have such a theory, and we may still be a long way from having one, but we do already know many of the properties that it must have. And we shall see in later chapters that we already know a fair amount about the predictions a quantum theory of gravity must make.

Atoms to Galaxies

In the first half of the twentieth century, physicists extended the reach of their theories from the everyday world of Isaac Newton to both the smallest and the largest extremes of our universe.

Now, if you believe that the universe is not arbitrary but is governed by definite laws, you ultimately have to combine the partial theories into a complete unified theory that will describe everything in the universe. But there is a fundamental paradox in the search for such a complete unified theory. The ideas about scientific theories outlined above assume we are rational beings who are free to observe the universe as we want and to draw logical deductions from what we see. In such a scheme it is reasonable to suppose that we might progress ever closer toward the laws that govern our universe. Yet if there really were a complete unified theory, it would also presumably determine our actions—so the theory itself would determine the outcome of our search for it! And why should it determine that we come to the right conclusions from the evidence? Might it not equally well determine that we draw the wrong conclusion? Or no conclusion at all?

The only answer that we can give to this problem is based on Darwin’s principle of natural selection. The idea is that in any population of self-reproducing organisms, there will be variations in the genetic material and upbringing that different individuals have. These differences will mean that some individuals are better able than others to draw the right conclusions about the world around them and to act accordingly. These individuals will be more likely to survive and reproduce, so their pattern of behavior and thought will come to dominate. It has certainly been true in the past that what we call intelligence and scientific discovery have conveyed a survival advantage. It is not so clear that this is still the case: our scientific discoveries may well destroy us all, and even if they don’t, a complete unified theory may not make much difference to our chances of survival. However, provided the universe has evolved in a regular way, we might expect that the reasoning abilities that natural selection has given us would also be valid in our search for a complete unified theory and so would not lead us to the wrong conclusions.

Because the partial theories that we already have are sufficient to make accurate predictions in all but the most extreme situations, the search for the ultimate theory of the universe seems difficult to justify on practical grounds. (It is worth noting, though, that similar arguments could have been used against both relativity and quantum mechanics, and these theories have given us both nuclear energy and the microelectronics revolution.) The discovery of a complete unified theory, therefore, may not aid the survival of our species. It may not even affect our lifestyle. But ever since the dawn of civilization, people have not been content to see events as unconnected and inexplicable. We have craved an understanding of the underlying order in the world. Today we still yearn to know why we are here and where we came from. Humanity’s deepest desire for knowledge is justification enough for our continuing quest. And our goal is nothing less than a complete description of the universe we live in.

4

NEWTON’S UNIVERSE

OUR PRESENT IDEAS ABOUT THE MOTION of bodies date back to Galileo and Newton. Before them, people believed Aristotle, who said that the natural state of a body was to be at rest, and that it moved only if driven by a force or impulse. It followed that a heavier body should fall faster than a light one because it would have a greater pull toward the earth. The Aristotelian tradition also held that one could work out all the laws that govern the universe by pure thought: it was not necessary to check by observation. So no one until Galileo bothered to see whether bodies of different weights did in fact fall at different speeds. It is said that Galileo demonstrated that Aristotle’s belief was false by dropping weights from the Leaning Tower of Pisa in Italy. This story is almost certainly untrue, but Galileo did do something equivalent: he rolled balls of different weights down a smooth slope. The situation is similar to that of heavy bodies falling vertically, but it is easier to observe because the speeds are smaller. Galileo’s measurements indicated that each body increased its speed at the same rate, no matter what its weight. For example, if you let go of a ball on a slope that drops by one meter for every ten meters you go along, the ball will be traveling down the slope at a speed of about one meter per second after one second, two meters per second after two seconds, and so on, however heavy the ball. Of course a lead weight would fall faster than a feather, but that is only because a feather is slowed down by air resistance. If you drop two bodies that don’t have much air resistance, such as two different lead weights, they fall at the same rate. (We will see why shortly.) On the moon, where there is no air to slow things down, the astronaut David R. Scott performed the feather-and-lead-weight experiment and found that indeed they did hit the ground at the same time.

Galileo’s measurements were used bv Newton as the basis of his laws of motion. In Galileo’s experiments, as a body rolled down the slope it was always acted on by the same force (its weight), and the effect was to make it constantly speed up. This showed that the real effect of a force is always to change the speed of a body, rather than just to set it moving, as was previously thought. It also meant that whenever a body is not acted on by any force, it will keep on moving in a straight line at the same speed. This idea was first stated explicitly in 1687, in Newton’s
Principia Mathematica,
and is known as Newton’s first law. What happens to a body when a force does act on it is given by Newton’s second law. This states that the body will accelerate, or change its speed, at a rate that is proportional to the force. (For example, the acceleration is twice as great if the force is twice as great.) The acceleration is also smaller the greater the mass (or quantity of matter) of the body. (The same force acting on a body of twice the mass will produce half the acceleration.) A familiar example is provided by a car: the more powerful the engine, the greater the acceleration, but the heavier the car, the smaller the acceleration for the same engine.

In addition to his laws of motion, which describe how bodies react to forces, Newton’s theory of gravity describes how to determine the strength of one particular type of force, gravity. As we have said, that theory states that every body attracts every other body with a force that is proportional to the mass of each body. Thus, the force between two bodies would be twice as strong if one of the bodies (say, body A) had its mass doubled. This is what you might expect, because one could think of the new body A as being made of two bodies, each with the original mass. Each of these would attract body B with the original force. Thus the total force between A and B would be twice the original force. And if, say, one of the bodies had six times the mass, or one had twice the mass and the other had three times the mass, then the force between them would be six times as strong.

You can now see why all bodies fall at the same rate. According to Newton’s law of gravity, a body of twice the weight will have twice the force of gravity pulling it down. But it will also have twice the mass and thus, according to Newton’s second law, half the acceleration per unit force. According to Newton’s laws, these two effects exactly cancel each other out, so the acceleration will be the same no matter what the weight.

Gravitational Attraction of Composite Bodies

If the mass of a body is doubled, so is the gravitational force that it exerts

Newton’s law of gravity also tells us that the farther apart the bodies, the lesser the force. The law says that the gravitational attraction of a star is exactly one-quarter that of a similar star at half the distance. This law predicts the orbits of the earth, the moon, and the planets with great accuracy. If the law were that the gravitational attraction of a star went down faster or slower with distance, the orbits of the planets would not be elliptical; they would either spiral into the sun or escape from the sun.

The big difference between the ideas of Aristode and those of Galileo and Newton is that Aristotle believed in a preferred state of rest, which any body would take up if it was not driven by some force or impulse. In particular, he thought that the earth was at rest. But it follows from Newton’s laws that there is no unique standard of rest. One could equally well say that body A was at rest and body B was moving at constant speed with respect to body A, or that body B was at rest and body A was moving. For example, if you set aside for a moment the rotation of the earth and its orbit around the sun, you could say that the earth was at rest and that a train on it was traveling north at ninety miles per hour or that the train was at rest and the earth was moving south at ninety miles per hour. If you carried out experiments with moving bodies on the train, all Newton’s laws would still hold. Is Newton right or is Aristotle, and how do you tell?

One test would be this: imagine you are enclosed in a box, and you do not know whether the box is resting on the floor of a moving train or on solid earth, the latter being the standard of rest according to Aristotle. Is there a way to determine which it is? If so, maybe Aristotle was correct—being at rest on the earth is special. But if you carried out experiments in your box on the train, they would turn out exactly the same as they would within your box on the "stationary" train platform (assuming no bumps, turns, or other imperfections in the train ride). Playing Ping-Pong on the train, you would find that the ball behaved just like a ball on a Ping-Pong table by the track. And if you are in your box and play the game at different speeds relative to the earth, say at zero, fifty, and ninety miles per hour, the ball will behave the same in all these situations. This is how the world behaves, and it is what the mathematics of Newton’s laws reflects: there is no way to tell whether it is the train or the earth that is moving. The concept of motion makes sense only as it relates to other objects.

Does it really matter whether Aristotle or Newton is correct? Is this merely a difference in outlook or philosophy, or is it an issue important to science? Actually, the lack of an absolute standard of rest has deep implications for physics: it means that we cannot determine whether two events that took place at different times occurred in the same position in space.

To picture this, suppose someone on a train bounces a Ping-Pong ball straight up and down, hitting the table twice on the same spot one second apart. To that person, the locations of the first and second bounces will have a spatial separation of zero. To someone standing beside the track, the two bounces would seem to take place about forty meters apart, because the train would have traveled that distance down the track between the bounces. According to Newton, the two observers have an equal right to consider themselves at rest, so both views are equally acceptable. One is not favored over another, as Aristotle had believed. The observed positions of events and the distances between them would be different for a person on the train and one beside the track, and there would be no reason to prefer one person’s observations to the other’s.

Newton was very worried by this lack of absolute position, or absolute space, as it was called, because it did not accord with his idea of an absolute God. In fact, he refused to accept the lack of absolute space, even though his laws implied it. He was severely criticized for this irrational belief by many people, most notably by Bishop Berkeley, a philosopher who believed that all material objects and space and time are an illusion. When the famous Dr. Johnson was told of Berkeley’s opinion, he cried, "I refute it thus!" and stubbed his toe on a large stone.

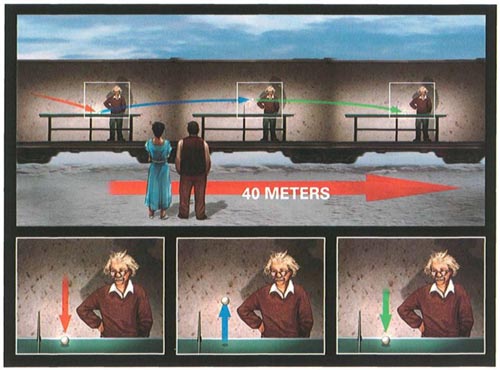
Relativity of Distance

The distance—and the path—that an object travels can look different to different observers.

Both Aristotle and Newton believed in absolute time. That is, they believed that one could unambiguously measure the interval of time between two events and that this time would be the same whoever measured it, provided the person used a good clock. Unlike absolute space, absolute time was consistent with Newton’s laws. And it is what most people would take to be the commonsense view. However, in the twentieth century physicists realized that they had to change their ideas about both time and space. As we will see, they discovered that the length of time between events, like the distance between the points where the Ping-Pong ball bounced, depends on the observer. They also discovered that time was not completely separate from and independent of space. The key to these realizations was new insight into the properties of light. They may seem counter to our experience, but although our apparently commonsense notions work well when dealing with things such as apples, or planets that travel comparatively slowly, they don’t work at all for things moving at or near the speed of light.