Why Beauty is Truth (21 page)
Read Why Beauty is Truth Online
Authors: Ian Stewart

After much effort, the University of Christiania was persuaded to grant Niels enough money for a research visit to Paris, where he would meet some of the world's leading mathematicians. In preparation for the trip, he decided that he needed printed copies of his best work. He believed that his impossibility proof for the quintic would impress his French peers; unfortunately, all of his work had been printed in Norwegian, in an obscure journal. He therefore decided that he should get his work on the theory of equations printed privately in French. Its title was “Memoir on algebraic equations, wherein one proves the impossibility of solving the general equation of the fifth degree.”
To save on printing costs, Niels distilled his ideas down to their essentials, and the printed version ran a mere six pages. This was a lot less than Ruffini's 500 pages, but there are occasions in mathematics where brevity can make ideas more obscure. Many of the logical detailsâwhich in this area were crucialâhad to be left out. The paper was a sketch, not a proof.
Niels introduced it by writing, “Mathematicians have generally been occupied with the problem of finding a general method for solving algebraic equations, and several have made attempts to prove the impossibility of it. I dare to hope, therefore, that mathematicians will receive favorably this article which has for its purpose to fill this lacuna in the theory of equations.” It was a faint hope. Though he succeeded in visiting some mathematicians in Paris and getting them to agree to look at his
paper, its reasoning was so compressed that most of them probably found it incomprehensible. Gauss filed his copy but never read itâwhen it was found after his death, the pages were still uncut.
Later, perhaps realizing his mistake, Abel produced two longer versions of his proof, giving more of the details. Having by this time heard of Ruffini, he wrote in these versions, “The first to attempt a proof of the impossibility of an algebraic solution of the general equation was the mathematician Ruffini; but his memoir is so complicated that it is difficult to judge the correctness of the argument. It appears to me that his reasoning is not always satisfactory.” But like everyone else, he didn't say
why.
Ruffini and Abel wrote their arguments in the formal mathematical language of the time, which was not well suited to the style of thinking required. Mathematics then was mainly concerned with specific, concrete ideas, whereas the key to the theory of equations is to think in rather general termsâabout structures and processes rather than specific
things.
Thus their ideas were difficult for their contemporaries to grasp for reasons that went beyond language. But even for modern mathematicians, using the terminology of the period would make comprehension difficult.
Fortunately, we can grasp the essential features of their analysis by employing an architectural metaphor. One way to think about Ruffini's almost-proof, and of Abel's complete proof, is to imagine building a
tower.
This tower has a single room on each floor, with a ladder that connects it to the room above. Each room contains a large sack. If you open the sack, millions of algebraic formulas spill out across the floor. At first sight, these formulas have no special structure and appear to have been harvested at random from the pages of algebra texts. Some are short, some long; some are simple, some extraordinarily complicated. A closer look, however, reveals family resemblances. The formulas in a given sack have lots of common features. The formulas in the sack in the room above have different common features. The higher we climb the tower, the more complicated the formulas in the sacks become.
The sack on the first floor, at ground level, contains all of the formulas that you can build by taking the coefficients of the equation and then adding them together, subtracting, multiplying, and dividing themâover
and over, as many times as you like. In the world of algebraic formulas, once you have the coefficients, all of these “harmless” combinations come along pretty much free of charge.
To climb the ladder to the floor above, you must take some formula out of the sack, and use it to form a
radical.
It might be a square root, a cube root, a fifth root, whatever. But the formula whose root you are taking must have come from that sack. You can always take it to be a
p
th root where
p
is prime, because more complex roots can be built from prime ones, and this simple observation is surprisingly helpful.
Whichever root you decide to take, when you arrive on the second floor, you find a second sack, whose contents are initially identical to those of the sack of the first floor. But you open the sack, and throw in your new radical.
Formulas
breed.
When Noah landed his ark on Mount Ararat, he told all the creatures inside it to go forth and multiply. The formulas in the sack do more than that: they go forth and multiply, add, subtract, and divide. After a few seconds of frenzied activity, the sack on the second floor is bulging with all possible “harmless” combinations of the coefficients of the equation
and
your new radical. Compared to the sack on the first floor, there are many new formulasâbut they all resemble each other; each of them includes your radical as a new component.
You do much the same to get to the third floor. Again you pick some formula from the new sackâjust oneâand form a new radical by taking some (prime) root of that formula. You carry your new radical up the ladder to the third floor, toss it in the sack, and wait for the formulas to carry out their mating rituals.
And so on. Each new floor introduces a new radical, and new formulas appear in the sack. At any stage, all of those formulas are built from the coefficients, together with any of the radicals introduced so far.
Eventually you reach the top floor of the tower. And you complete your questâto solve the original equation by radicalsâprovided that, tucked away inside the sack in the attic, you can find at least one root of that equation.
There are many conceivable towers. They depend on which formulas you choose, and which radicals you take. Most fail dismally, and no hint of the desired root can be found. But if the quest is possible, if some formula built from successive radicals yields a solution, then the corresponding tower does indeed have a root in its attic. For the formula tells us
exactly how to obtain that root by adjoining successive radicals. That is, it tells us exactly how to build the tower.
We can reinterpret the classic solutions of the cubic, the quartic, and even the Babylonian solution of quadratics in terms of these towers. We begin with the cubic, because this is complicated enough to be typical, but simple enough to be comprehensible.
Cardano Tower has only three floors.
The sack on the first floor contains the coefficients and all of their combinations.
The ladder to the second floor requires a square root. A very particular square root, that of a specific formula in the first sack. The sack on the second floor contains all combinations of this square root, together with the coefficients.
The ladder to the third floor, the attic, requires a cube rootâagain, a specific one. It is the cube root of a particular formula involving the coefficients and the square root that you used to reach the floor below. Does the sack in the attic contain a root of the cubic equation? It does, and the proof is Cardano's formula. The ascent of the tower is a success.
Ferrari Tower is taller; it has five floors.
The first floor, as always, has a sack that contains just the combinations formed by the coefficients. You reach the second floor by forming harmless combinations and then taking a suitable square root. You reach the third floor by forming harmless combinations and then taking a suitable cube root. You reach the fourth floor by forming harmless combinations and then taking a suitable square root. Finally, you clamber up to the fifth floorâthe atticâby forming harmless combinations and then taking a suitable square root.

Solving the quadratic, cubic, and quartic.
And now, the sack in the attic does indeed contain what you are seeking, a root of the quartic equation. Ferrari's formula provides the instructions for building precisely such a tower.
The Tower of Babel, which solves the quadratic, also fits the metaphor. But it turns out to be a stumpy tower with only two floors. The sack on the first floor contains just the combinations of the coefficients. A single carefully chosen square root conducts you to the floor above, the attic. Inside that sack is a root of the quadraticâin fact, both of them. The Babylonian procedure for solving quadratics, the formula you were taught at school, tells us so.
What about the quintic?
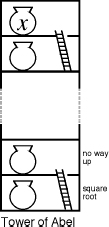
Suppose that a formula to solve the quintic by radicals really does exist. We don't know what it is, but we can infer a lot about it nonetheless. In particular, it must correspond to some tower. Let me call this hypothetical tower the Tower of Abel.
The Tower of Abel could contain hundreds of floors, and its ladders may involve all sorts of radicalsâ19th roots, 37th roots, we don't know. All we know for sure is that the sack on the first floor contains just the harmless combinations of coefficients. We fondly imagine that up in the attic, above the clouds, is a sack containing some root of the quintic.
We ask how to climb the tower, and the mathematics tells us that there is only one way to get to the second floor. We have to take one particular square root. There is no other way up.