Warped Passages (62 page)

To understand how this works, suppose that, like most people (at least those who haven’t read this book), you were completely ignorant of the fifth dimension—which is, after all, invisible. Untroubled in the belief that you live in four dimensions, you would know only about four-dimensional gravity, which you would believe was communicated by a conventional four-dimensional graviton. In the four-dimensional effective theory that describes what you see, there would be only one gravitational force, and there could therefore be only a single type of four-dimensional graviton. All particles would interact with that single type of graviton. But that graviton wouldn’t contain any information about the location of a particle in the original, higher-dimensional theory.

This reasoning makes it look as though all graviton interactions should be the same—that is, independent of where in the fifth dimension an object originated. After all, you wouldn’t know that the object originated in the fifth dimension, or even that there
was
a fifth dimension. Newton’s constant of gravitation, which determines the graviton’s interaction strength, would be the single quantity that determines the strength of all four-dimensional gravitational interactions. But in the previous section, we saw that gravity’s interactions are weaker as you moved from the Gravitybrane towards the Weakbrane. This leaves the question, how can gravity’s strength encompass information about an object’s fifth-dimensional location?

The resolution to the apparent paradox hinges on the fact that gravitational attraction is also proportional to mass, and mass at different points along the fifth dimension can and must be different. The only way to reproduce the weakened graviton interaction on each successive slice along the fifth dimension is to measure mass differently on each four-dimensional slice.

One of the many remarkable properties of warped spacetime is that as you move from the Gravitybrane to the Weakbrane, energies
and momenta shrink. The shrinking energies and momenta (and consistency with quantum mechanics and special relativity) also tell us that distance and time must expand (as shown in Figure 82). In the geometry I am describing, size, time, mass, and energy all depend on location. Four-dimensional sizes and masses inherit values that depend on their original five-dimensional positions. Physics looks four-dimensional. But the ruler with which length is measured, or the scale with which mass is measured, depends on the original five-dimensional location. Residents of the Gravitybrane or the Weakbrane both see four-dimensional physics, but they would measure different sizes and expect different masses.

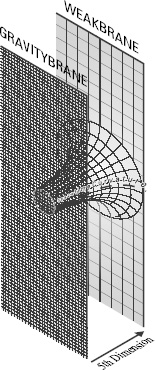
Figure 82.
Sizes increase (and masses and energies decrease) as one moves from the Gravitybrane to the Weakbrane.

The gravitational attraction of masses of particles that originate further away from the Gravitybrane in the original five-dimensional theory is smaller in the four-dimensional effective theory because the
masses themselves are smaller. This is because at each position in the fifth dimension, mass and energy get
rescaled
by an amount proportional to the amplitude of the graviton’s probability function at that particular point. And the
warp factor
, which is the amount by which you rescale the energies, is smaller further away from the Gravitybrane. In fact, its plot has exactly the same shape as the graviton’s probability function. Masses and energy therefore shrink by a different factor at every point along the fifth dimension—and the warp factor determines by how much.

This rescaling might seem arbitrary, but it’s not. It is subtle, however, so let’s first consider an analogous situation. Suppose that we were to measure time in terms of how long it takes to travel 100 km by train. I will call these units of time TT (train time) units. This is a fine measure of time, except that your determination of time would depend on where you are traveling: are the trains fast there or are they slow? For example, suppose that a movie lasted two hours. If an American train took an hour to travel 100 km, an American viewer would cover 200 km over the course of that movie and say that the movie lasted 2 TTs. A French viewer riding the TGV, on the other hand, would think that the movie lasted 6 TTs, because express trains in France travel about three times faster and the French viewer would need to watch their DVD during a 600-km-long train ride to see how it ends. Because the French viewer’s train covers 100 km in 20 minutes, whereas an American’s train covers the same distance in an hour, you need to rescale train time if Americans and French are to share common units and agree on the TT length of the movie. To convert from French to American time, you would have to rescale the French train time by a factor of three.

Similarly, on the Weakbrane, where the graviton interaction is far smaller than on the Gravitybrane, the units for the scale used to measure energy must be rescaled to take account of gravity’s weakness. At the Weakbrane, the rescaling is by an enormous amount, 10
¹⁶
, ten million billion. What this means is that whereas on the Gravitybrane all fundamental masses are expected to be
M
_Pl
(the Planck scale mass), on the Weakbrane they are expected to be only about 1,000 GeV, a factor of 10
¹⁶
smaller. Masses of new particles that live on the Weakbrane might be somewhat larger, perhaps 3,000
or 5,000 GeV, but they shouldn’t be much larger than that since all masses have been rescaled enormously.

The hierarchy problem arises when all masses get raised to the largest mass around. If that mass is the Planck scale mass, all masses are expected to be about as big as the Planck scale mass. But owing to the rescaling, if you originally thought that the Planck scale mass was the expected mass for everything on the Gravitybrane, then on the Weakbrane you would conclude that a TeV, sixteen orders of magnitude smaller, is the expected mass.
^*
This means that the mass of the Higgs particle is not at all disturbing: a mass of about a TeV—ten million billion times smaller than the Planck scale mass—is expected, even though gravity is weak. The rescaling, which is essential in this interpretation, solves the hierarchy problem.

By the same reasoning, all new objects on the Weakbrane, including strings, should have mass of about a TeV. This tells us that this model could have dramatic experimental consequences. On the Weakbrane, the extra particles associated with strings would be very much lighter than those on the Gravitybrane—or in a four-dimensional world, for that matter. The Weakbrane presents a fabulous scenario from the perspective of discovering extra dimensions. If this idea is correct, then low-mass particles from extra dimensions should be near at hand. TeV-mass particles would abound on the Weakbrane.

Everything on the Weakbrane is expected to be lighter than the Planck scale mass by a factor of 10
¹⁶
. And according to quantum mechanics, smaller mass means larger size. Athena’s shadow would grow as she went from the Gravitybrane towards the Weakbrane. This tells us that strings on the Weakbrane should not be 10
^-33
cm in size. Instead, they should also be sixteen orders of magnitude larger—that is, about 10
^-17
cm.

Although I have focused on a two-brane scenario with a specific warp factor, the features we have considered are likely to be more general than this particular example. With extra dimensions there is
good reason to expect disparate masses. The particle physics intuition that masses should be more or less the same is violated, and a wide range of masses is
expected
. Particles located in different locations would naturally have different masses. Their shadows change as you move around. In our four-dimensional world, the result would be a range of sizes and masses, and that is what we observe.

Further Developments

When our paper explaining the hierarchy in terms of warped geometry appeared in 1999, most of our colleagues didn’t recognize that it was a genuinely new theory, very different from the large dimensions idea. Joe Lykken said to me, “Reaction built slowly. Eventually everyone understood that this paper [and another that Chapter 22 will describe] were big and new and generic and opened a whole new arena of ideas, but not at first.”

For months after our paper came out, I was asked to give talks about my work on “large extra dimensions.” I kept having to object that the beauty of our theory is precisely that the dimensions are not large! In fact, Mark B. Wise, a (very aptly named) Caltech particle theorist, laughed at the title I was assigned for a plenary talk in the closing session of the Lepton-Photon Conference of 2001, the major particle conference where experimenters present important results. The organizers had given my talk a title that referred to all research on extra dimensions except my own!

Mark and his then student, Walter Goldberger, were two of the first to understand the merits of the warped scenario. But they also recognized that Raman and I had left a potential gap in our result that needed to be filled. We had assumed that brane dynamics would naturally lead to branes that are a modest distance apart. However, we had not explicitly said how the distance between the two branes is established. This wasn’t just a detail; our theory’s role as a solution to the hierarchy problem depended on being able to readily stabilize the two branes a small but finite distance apart. It was possible that the inverse exponential function of the distance (which we wanted to be extremely tiny), rather than the distance itself, would turn out
naturally to be a modest number. If so, the predicted hierarchy between the weak scale mass and the Planck scale mass would be a modest number, and not the (much smaller) inverse exponential of that number—and our solution wouldn’t work.

Goldberger and Wise did the important research that closed this potentially treacherous loophole in the theory Raman and I had presented. They demonstrated that the distance between the two branes is a modest number, and the inverse of the exponential of that distance is extremely tiny, exactly as was required for our solution to work.

Their idea was elegant, and turned out to be of more general validity than anyone realized at the time. As it happens, any stabilization model is very similar to theirs. Goldberger and Wise suggested that in addition to the graviton, there was a massive particle that lived in the five-dimensional bulk. They assigned properties to this particle that made it act like a spring. In general, a spring has a favored length; any larger or smaller length would carry energy that would make the spring move. Goldberger and Wise had introduced a particle (and associated field) for which the equilibrium configuration for the field and the branes would involve a modest brane separation—again, just what our solution to the hierarchy required.

Their solution relied on two competing effects, one that favors widely separated branes and another that favors nearby branes. The result is a stable compromise position. The combination of the two counteracting effects leads naturally to a two-brane model in which the two branes are a moderate distance apart.

The Goldberger-Wise paper made it clear that the warped two-brane scenario really was a solution to the hierarchy problem. And the fact that the separation between the branes could be fixed was important for another reason. If the distance between the branes was undetermined, the branes could move closer together or further apart as the temperature and energy of the universe evolves. If the brane separation could change, or if different sides of the five-dimensional universe could expand at different rates, the universe would not evolve in the way it’s supposed to in four dimensions. Since astrophysicists have tested the expansion of the universe late in its evolution, we know that recently the universe has expanded as if it were four-dimensional.

With the Goldberger-Wise stabilization mechanism, the warped five-dimensional universe agrees with cosmological observations. Once the branes are stabilized with respect to each other, the universe would evolve as if it were four-dimensional, even if it actually has five dimensions. Even though there would be a fifth dimension, the stabilization would rigidly constrain different places along the fifth dimension so that they would evolve in the same way, and the universe would behave as it would in four dimensions. Since the Goldberger-Wise stabilization should happen relatively early on, the warped universe would look four-dimensional for most of its evolution.

Once stabilization and cosmology were understood, the warped geometry solution to the hierarchy problem was in business. Many other interesting developments about this warped geometry soon followed. One of these was unification of forces. All forces, including gravity, might be unified at high energies in the warped geometry we’re considering!

Warped Geometry and Unification of Forces

Chapter 13 explained how a major feather in the cap of supersymmetry is that it can successfully accommodate the unification of forces. Extra-dimensional theories that addressed the hierarchy problem seemed to forfeit this potentially important development. Since we have not seen any conclusive experimental evidence for unification, such as proton decay, this is not necessarily a major loss, as we don’t yet know for certain that unification is correct. Nonetheless, three lines meeting at a point is intriguing and might presage something meaningful. Even if unification is not yet firmly established, we shouldn’t abandon it too hastily.