Warped Passages (75 page)

24
. The trajectory of a particle is a worldline that gives the particle’s position as a function of time. The trajectory of a string is a surface that describes the position of the entire string as it moves through time. The worldsheet represents the motion of an open string, whereas the worldtube represents the motion of a closed string. This is shown in Figure M3, which illustrates the motion through time and the “softer” interactions of strings.

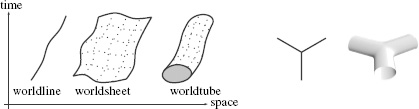
Figure M3.
(Left figure) Wordline of a particle, worldsheet of an open string, worldtube of a closed string. (Right figure) Interactions of three particles and three strings.

25
. The string tension is not always as high as you would guess from the Planck scale energy. It depends on how strongly strings interact. Joe Lykken and others have considered the possibility that it is much smaller, in which case the additional particles from string theory could be much lighter.

26
. Actually, according to the duality we learn about in this chapter, even the probes used to study a given version of string theory change character
when the coupling becomes strong. So if Ike really was part of the string world, he, too, would change.

27
. They can also extend in zero dimensions, in which case they are new kinds of particle called Do-branes, or in one dimension, in which case they are new types of string called D1-branes.

28
. Branes don’t necessarily interact via ordinary charges. They interact via a higher-dimensional generalization of charges.

29
. The symmetry actually rotates branes into each other, but this is beyond the technical reach of this book (and would make Igor’s head spin).

30
. Ordinarily, the gaugino masses fall in a ratio of about 1:3:30, where the photino is the lightest, winos are next (though the zino might be a little heavier or lighter than the winos), and the gluinos are heaviest. In sequestered models the ratio is 1:2:8, where the winos are the lightest, the photino is heavier, and the gluino is again the heaviest.

31
. The wavefunctions of the Kaluza-Klein modes are the modes that occur in the generalized Fourier decomposition of the higher-dimensional wavefunction.

32
. This also assumes that there are no singularities in the spacetime geometry—that is, no place where the space shrinks to zero size.

33
. D. Cremades, S. Franco, L. Ibanez, F. Marchesano, R. Rabadan, and A. Uranga also suggested an interesting alternative. Their idea is that particles are not confined on an individual brane, but are instead confined to the intersections of multiple branes. As with separated parallel branes, strings extending between branes will generally be heavy. But light or massless particles arise from zero-length strings, which in this case would be confined to the region where the branes intersect.

34
. We can also show this in a slightly different way with a more mathematical argument. When there are curled-up dimensions, the force lines emanating from a massive object behave according to the gravitational law of the higher-dimensional theory at short distances and according to four-dimensional gravity at long distances. The only way to reconcile the two force laws and switch smoothly from one to the other is by noting that at about the distance corresponding to the extra dimensions’ sizes, the force lines spread as if there were only four dimensions, but with a reduced strength because of the extra volume of the curled-up space. Beyond the size of the extra dimensions, gravity behaves four-dimensionally but with its strength suppressed by the spreading out over the extra-dimensional volume.

Newton’s law of gravitation says that when there are three spatial dimensions, the force is proportional to 1/
M
_Pl
²
× 1/
r
²
. If there are
n
additional
dimensions, the force law would be 1/
M
<sup>n
+ 2</sup>
× 1/
r
<sup>n
+ 2</sup>
, where
M
sets the strength of higher-dimensional gravity, similarly to the way in which
M
_Pl
sets the strength of four-dimensional gravity. Notice that the higher-dimensional force law varies more quickly with
r
since the force lines would spread over a hypersphere whose surface would have
n
+ 2 dimensions (as opposed to the two-dimensional surface of a sphere that gives rise to the force law of three-dimensional space). However, when the extra-dimensional volume is finite and the
n
extra dimensions have size
R
, the force law will be 1/
M
<sup>n
+ 2</sup>
× 1/
R
ⁿ
x 1/
r
²
when
r
is greater than
R
, and the force lines can no longer spread in the extra dimensions. This is the form of a three-spatial-dimensional force law if we make the identification
M
_Pl
²
=
M
<sup>n
+ 2</sup>
R
ⁿ
. Since
R
ⁿ
is the volume of the higher-dimensional space, we find that the strength of gravity decreases with volume, or equivalently (because gravity’s strength is weaker when the Planck scale energy is bigger), the Planck scale energy is big if the volume is big.

35
. A flat metric with three spatial dimensions is
ds
²
=
dx
²
+
dy
²
+
dz
²
+
c
²
dt
²
. Because there are no spatial or time-dependent coefficients, measurements are independent of where you are or which direction you point in; that is to say, spacetime is completely flat. All three spatial coordinates as well as the time coordinate (up to the minus sign that always singles time out) are treated the same; that is, the coefficients of the terms in the metric are completely independent of time and spatial location.

36
. The metric in the warped geometry is
ds
²
=
e
^-
k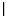
r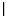
(
dx
²
+
dy
²
+
dz
²
+
c
²
dt
²
) +
dr
²
, where
r
is the coordinate of the fifth dimension. That tells us that at any fixed location in the fifth dimension, which corresponds to fixed
r
, spacetime is completely flat. However, the overall
r
-dependent factor tells us that how we measure size changes according to the position of an object in the fifth dimension. The exponential falloff of the coefficient, which is the warp factor, is the reason that the graviton’s probability function falls exponentially, and is also why we need to rescale mass, energy, and size to make a single, four-dimensional effective theory.

37
. Because space is not flat, the extra-dimensional volume that enters when we calculate
M
_Pl
in four dimensions is not simply
M
_Pl
³
R
, as it would be when space is flat. Instead, the value of
M
_Pl
depends on the curvature. If the metric has the form
ds
²
=
e
^-
k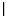
r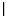
(
dx
²
+
dy
²
+
dz
²
−
c
²
dt
²
) +
dr
²
, where
r
is the coordinate of the fifth dimension, then, roughly,
M
_Pl
²
=
M
³
/
k
. In other words, the size of the space is largely irrelevant. This makes sense because the curvature of space—not the extra dimension’s size—determines how the field lines spread in the extra dimension and hence the strength of four-dimensional gravity. In fact, there is small dependence on
R
: the real formula is
M
_Pl
²
=
M
³
/
k
(1 −
e
^-kR
), but when
kR
is big, the exponential term is largely irrelevant and can be neglected.