My Time in Space (20 page)

In the mouth of Gardner (whom the anarchic philosopher of science Paul Feyerabend has called ‘the pit-bull of scientism’), ‘counter-cultural’ would be a term with bite. What had urged him to this attack? To my amazement I found a letter of
Gardner’s
, of slightly earlier date, quoted on two web sites including the Math Forum discussion group, saying that he had proposed to write a column on Spencer-Brown but had been dissuaded by another mathematician, Donald Knuth, on the grounds that to do so would give publicity to a charlatan! What could this work be, that seemed to have provoked a move to suppress it? Although I had little confidence in my ability to judge it, I was determined to look into it.

The only available account by Spencer-Brown of his
controversial
proof of the four-colour theorem, I gathered from the LoF site, was in an English appendix to a German edition of
Laws
of
Form,
to be had from a company called Astro. When I located Astro’s website I was discouraged to find it largely concerned with astrology and sexual magic. In any case, they failed to respond to
my e-mails, and so, with some trepidation (one Internet source having described him as ‘a dreadful curmudgeon but at the same time one of the most charming, humorous, and delightful people I have ever met’), I wrote to the author himself. I was hoping for some closure to the story of high intellectual endeavour I wanted to tell and of which I had already written the earlier part; it would have been satisfying to see the new proof victorious over all doubters, so that the conclusion of my essay could carry it in
procession
as Cimabue’s new Madonna was carried by the admiring townsfolk of Florence. Instead, I have become, briefly, the Fool of pelting storms, too fraught with personal matters to be recounted here, far out on a conceptual heath. How simple it would be to round off this adventure if Spencer-Brown and his proof were, ‘not in lone splendour hung aloft the night’, but
glittering
away up there as unquestionable constellations with Pascal and his mystic hexagram! But I have discovered, with pain, that the realm of pure ideas is one of the battlefields of human affairs, of which we will never have a colourable map.

A week or so after the date of my letter, the telephone rang: George Spencer-Brown himself, from his new address in the West Country. He began with a long complaint that the telephone number on my letterheading did not include the international dialling code, continued with some disobliging remarks on a little book I had sent him on prime numbers by a friend of mine, and half an hour later he was still telling me about his own
astounding
discoveries in the theory of prime numbers, including an unpublished proof of Goldbach’s conjecture. I arranged to send him a cheque for the price of the German edition of
Laws
of
Form,
which arrived a few days later together with a photocopy of a long and rather bizarre-looking handwritten paper on prime numbers,
and a twenty-page letter in which he dealt at length with the dialling-code question again, again abused my friend’s book, went into a rant against G.H. Hardy and
his
book, (‘a [mediocre]
mathematician’s
apology [for being mediocre]’), broke off to include a poem ‘In Praise of Lying’ by Richard de Vere, presumably a
pseudonym
of Spencer-Brown himself, got into his stride by
remarking
that if he is let loose in any mathematical discipline, he will either abolish it or transform it, and ended with a rich account of his dealings with God, Sir Stanley Unwin and Bertrand Russell over the publication of
Laws
of
Form.

Several parts of this inordinate communication infuriated me, and I wrote a riposte calculated to raise blisters on a battleship, but did not put it in the post because at the same time I was reading the new work on the four-colour theorem and finding it full of wonders. Instead, I devised a challenge, in a logical format that recurs in Spencer-Brown’s own writing:

His reply completely ignored this provocation; without even a preliminary ‘Dear Tim’ it opened with the claim that the only two great mathematicians of the twentieth century were
Ramanujan and himself, and that while Ramanujan was probably the cleverer I would already know that Spencer-Brown was the deeper. What was I to make of this? If false, the boast was absurd; if true, then it is to be expected that he would know it to be so, and it would be legitimate, if unconventional, to proclaim it. The question added to the interest of the journey I made to visit him, as a gesture of friendship and concern, in the summer of 2000.

I found Spencer-Brown to be a vigorous seventy-odd-
year-old
, with the fine domed head one associates with the
conventional
image of genius, and combative bushy eyebrows. He is still extremely productive – the floor of his cottage was ankle-deep in calculations – but in cramped circumstances, having held no
academic
post since the early 1980s when he was Visiting Professor of Mathematics at the University of Maryland, because, he claims, of the sort of calumny I had come across on the Internet. He has been denied outlet for his papers other than as appendices to
successive
editions of
Laws
of
Form
–
a shame, this, because it is a
classic
text, and in its current form suffers as a Palladian villa would from disproportionate extensions and ad hoc lean-to sheds. His conversation was challenging (we disagreed on the fundamental nature of reality, because, he said, I was ‘unenlightened’ – which remark enlightened me instantly to the fact that Buddhism can be as oppressive as any other system of belief), amusing (‘Most cats think the way to play chess is to knock the pieces off the board and chase them around the floor; but my kitten, having spent up to two hours sitting on my shoulder while I analyze a chess
position
, knows how to put out its paw and gently push a bishop from one square to another’), sometimes profound (‘To write a great book one must love one’s reader’), but unremittingly self-centred, so that after a few hours my own ego was struggling for oxygen.
When I picked up the phone to call a taxi, I found that the spiral flex between handset and base was so intricately twisted that I could only prise them a few inches apart, which made speaking difficult. During our subsequent telephone conversations I have often thought of that flex, for they have been extremely tangled and contorted. Calls would go on for an hour or more, leaving me sometimes with a valuable insight into Spencer-Brown’s mathematics and sometimes with a burden of history, too personal in relation to himself and libellous in relation to his fellow
professionals
(‘pipsqueaks’, the most of them) to be anything other than an obstacle to my writing of this account, and on several occasions ending in as much physical violence as can be transmitted by
telephone
.

He had some unfair advantages, I felt, in these bouts: an
extraordinarily
quick intelligence; an unshakeable belief in the
correctness
of whatever he was saying, even if, or especially if, it was the exact opposite of what he had said last time; and an utter
ruth-lessness
as to my
amour-propre.
Sometimes I tried to hang up on him, but he was always quicker on the draw and while I was still aiming a parthian shot I would hear the decisive click of his receiver. Latterly I undertook such exchanges with the enthusiasm I might have for running before the bulls of Pamplona, in
expectation
of an exhilarating scamper ending with a high probability of being trampled and gored. The relationship terminated, as M had predicted, with my dismissal into the category of pipsqueak. I had written asking for elucidation of certain steps that left me doubtful in two of his proofs of the four-colour theorem; there was no reply, and I accepted the break in this overheating
correspondence
with some relief, but eventually, since I needed
guidance
in order to progress with the mathematics, I telephoned him.
A useful little tutorial followed, but when I showed a reluctance to follow him in a death-defying leap from a result in
Laws
of
Form
to the obvious truth of the four-colour theorem he told me that if I couldn’t see the connection, after the thousands of poundsworth of free tuition he’d given me, I was an idiot. Like all the mathematicians who couldn’t or wouldn’t read his work, I just wasn’t good enough. And he rang off. I rang back instantly to remind him that despite his rudeness I would be writing about him, and was finally annihilated by another roar of ‘You’re not good enough!’

So, the message of all that sound and fury is:
Caveat
lector,
any idiocies in the following sketch of Spencer-Brown’s reformulation of the theory of maps are my own. In any case I can hardly go beyond the opening moves here, but I hope to convey something of the finesse of his approach. I begin by quoting the introduction to his own presentation:

A map, of the abstract sort we are considering, consists of a number of regions separated by borders, drawn on a flat surface; we count the area one would normally think of as outside the map (as the sea is outside a map of Ireland) as one of its regions. The points where borders meet are called nodes. Adjacent regions are those that share a border (not just a node). The shapes and sizes of the regions and borders are irrelevant; we can stretch and bend them as convenient. The first step is to standardize the map so that just three borders meet at any one node, as in Fig. 19. If the
four-colour
theorem is true for standard maps it must be true for all maps; so from here on we need only consider standard maps.

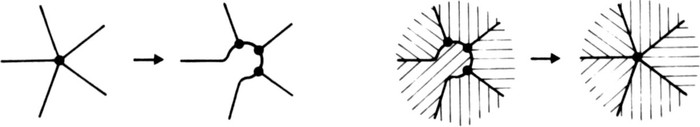
Fig. 19.
Left:
Eliminating a node at which more than three regions meet by
expanding
one of them.
Right:
If the map containing this new configuration can be four-coloured, so can an otherwise identical map containing the old configuration.

Suppose we have a map that can be coloured (so that no two adjacent regions are of the same colour – and from now on we will take this clause to be understood) with just four different colours. An economizing printer looking at this as a production problem might hit on the idea of using a red and a blue ink, say, plus a
purple
made by overprinting red with blue (or vice versa), leaving the white paper to represent the fourth colour; this is the format:
r
, or
b,
or both, or neither. Thus the four-coloured map could be printed from two plates, one of a number of red shapes and the other of a number of blue shapes – i.e. the map can be
factored
into two nodeless maps each of two colours (red and white, or blue and white), rather as the composite number 15 can be factored into the prime numbers 3 and 5 (Fig. 20). Spencer-Brown’s strategy is to prove that
any
standard map can be so factored – from which it
follows
that any map can be coloured with just four colours.